Roger Ayats defends his thesis on coherent structures in the transition to turbulence
Apr 25, 2022
En Roger Ayats defended his thesis co-directed by l’Alvaro Meseguer and Fernando Mellibovsky on April 22nd at Campus Nord. The thesis, which is entitled "Exact coherent structures in the transitional regime of shear and centrifugal flows", studies the transition mechanisms in shear and centrifugal flows by computing and analysing the role of exact coherent structures.
Turbulence is one of the major concerns for most technological problems involving fluid motion. Specially in aeronautics, a turbulent boundary layer results in structural stresses, vibrations and higher aircraft drag, leading to a significant increase in fuel consumption. Therefore, trying to comprehend the origin of turbulence by studying its most common transition routes is a crucial first step towards its effective control.
Transition to turbulence of an homogeneous flow is frequently mediated by transient visits to highly nonlinear laminar coherent structures that usually are at the threshold between laminarity and turbulence. From a dynamical systems point of view, these structures are invariant sets in the infinite- dimensional Navier-Stokes phase space that here we aim to identify in different canonical flows. With the use of direct numerical simulations together with Newton-Krylov solvers and Arnoldi iteration method for the linear stability analysis, invariant sets such as equilibria, relative equilibria or periodic orbits are accurately computed and tracked along the parameter space to understand the transition mechanisms. From a mathematical perspective, dynamical systems and bifurcation theory provide the suitable framework to understand the hydrodynamic instabilities and transition to turbulence from a deterministic point of view. In addition, the use of spectral methods for the spatial discretisation is particularly convenient due to the exponential convergence of the numerical solutions.
In the first work, the onset of transition in two-dimensional Plane Poiseuille flow is analysed. A new family of Tollmien-Schlichting waves, breaking the usual half-shift and reflect symmetry of the classical ones, has been identified and tracked across parameter space. In addition, the role of another classical travelling wave family that did not participate in the localisation mechanisms has been clarified.
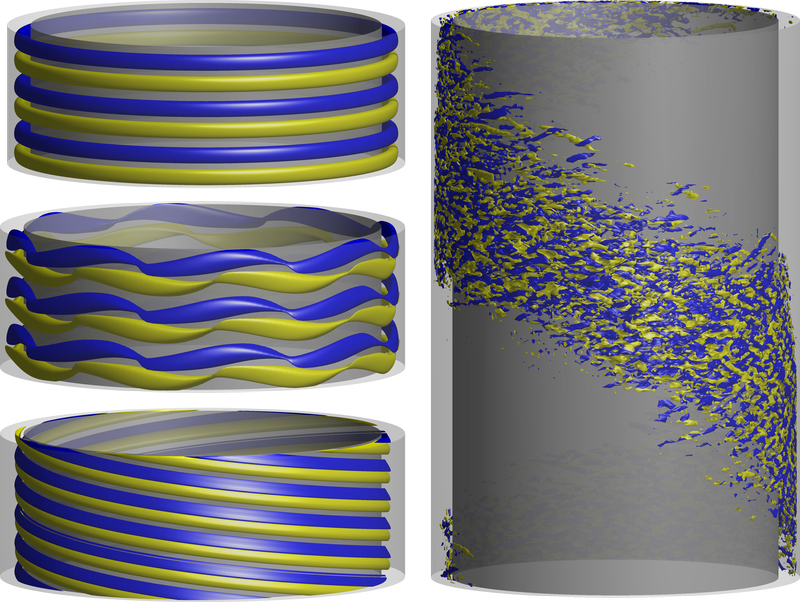
We continue by analysing the nonlinear mode competition in purely hydrodynamic and also magnetised Taylor-Couette flow. Finite-amplitude mixed-mode solution branches, arising from both purely hydrodynamic and magneto-rotational instabilities, are identified. These nonlinear mode interactions are efficiently computed in suitable skewed computational domains instead of the classical orthogonal ones, allowing for a significant reduction of the required computational resources.
Finally, the generalisation of extensional flows between biorthogonally stretching-shrinking parallel plates is analysed. Under the assumption of the self-similar ansatz, three-dimensional steady equilibria of the Navier-Stokes equations are identified and systematically tracked in parameter space, to cover all possible configurations of the acceleration rates and thus unfold all occurring bifurcations. After the explorations, up to seven different families of steady solutions have been identified, some of them related in pairs with symmetries. When increasing wall acceleration rates, the solution branches interact by means of fold and codimension-2 cusp bifurcations, increasing the complexity of the topology of equilibria.
Besides the specific interest attached to each one of the three problems we have addressed, these have further served as a proof-of-concept for the applicability and suitability of the methods and tools developed in the course of this thesis, which may assist in tackling a vast range of problems across a huge variety of physics and engineering disciplines.
Share: